Average Velocity formula for equal time intervals (with derivation)

In this post, we will derive the Average Velocity Formula for equal time intervals.
In this specific case, a body travels multiple distance intervals between the starting point and end point with different velocities, but taking equal time for every distance interval. For this case, we need to find out the average velocity formula.

Let’s see what this actually means and what is the formula.

Say, an object is moving from A to B (see Figure 1). The distance between A and B = AB = L meter

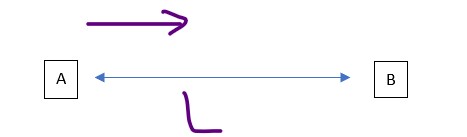
Say, this total distance L is made of n number of distance intervals like l₁,l₂, l₃, …and l_n.

So, we can write L = l₁ + l₂ + l₃ … + l_n ………………………. (1)

Say the object passes these distances with different velocities like v₁, v₂, v₃,…., and v_n. As said by the problem statement the distance intervals take equal time (say t) to pass through by the moving body.
So total time required to cross the entire distance T = n t ………. (2)

Now, if we rewrite the equation (1) in terms of velocities and time, then it becomes:
L = l₁ + l₂ + l₃ … + l_n
=> L = v₁t+ v₂t + v₃t … + v_nt
=> L = t (v₁+ v₂ + v₃ … + v_n) …………….. (3)

Now, from equations (3) and (2) let’s find out the equation of the Average Velocity for equal time intervals.

v_avg = L/T = t (v₁+ v₂ + v₃ … + v_n) / nt = (v₁+ v₂ + v₃ … + v_n) / n ………….. (Derived)

When n=2,

v_avg = (v₁+ v₂)/2